The Number Landscape Project
We propose the development of three large outdoor stations exploring the multiplicative properties of the natural numbers: the concepts of commutativity, prime, prime factorisation (and its uniqueness), and of the lowest common multiple of two numbers, extended to signed integers, standard topics in middle school curricula. Each is served by a small-scale version which prepares the visitor for the whole-body experience out of doors. No further captions are attached to the large versions: visitors can repeat some of the things they’ve already done on the small scale, or explore the objects in a freer way.
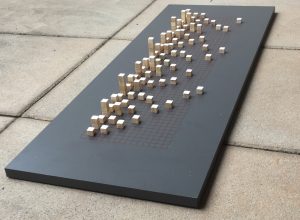
Number City
The model uses 1 cm cubes but we envisage this scaled by 50, so we need to allow a site 40 m by 10 m. The blocks will be in a white polymer but securely anchored to the ground. The surface between may be grassed. On this scale, the tallest block will be 2.50 m. There will be no labelling on the blocks. There will be no restriction on children climbing on them.
The fiction is that we’re looking at a city laid out along a waterside.
A counting strip runs from 1 (lower left) to 60 (upper right), the only concession to mathematics. Parallel to the water are blocks whose heights are the exponents of the primes increasing in sequence left to right. Thus one can read the prime factorisations of the numbers as one walks away from the water.
There are (at least) three significant observations:
- Walking from lower left to upper right, if you turn from the block you meet, you have an uninterrupted view to the water.
- There are no double blocks parallel to the water.
- You can identify rectangular plots (ground plans) which repeat parallel to the water, regardless of the blocks’ individual heights.
Look at these preparatory indoor tasks:
and for older students:
The Prime Climb Helix
What is shown is a half-scale version of what we envisage: a column 2.5 m high with a diameter of 10 cm in a white polymer. The numbers are screen-printed. The steel base has holes through which the ribbons hang to keep them separate. It is set in concrete. Several bolts secure the column to the base so that it cannot easily be detached.
Numbers run up the column in a helix from 1 to 150. Primes are marked in red, composite numbers in black. Because one circuit consists of 6 numbers, primes other than 2 and 3 are confined to two columns so that, if you view the column from the right direction, you see all the primes bar two.
There are seven coloured ribbons, one for each of the primes 5, 7, 11, 13, 17, 19. The task is to wind the ribbon of your choice down through all the multiples from the highest possible, to which it is attached, down through all lower ones, and anchor it to 0. Having done so for 5 and 7, the visitors find they cross on 35, thus knocking out a candidate prime from the special columns, and similarly for the other possible ribbon combinations. (Experimenters can consider what is special about 25, 49, 121.)
Out of hours the ribbons are removed for safe keeping.
Look at this preparatory indoor task:
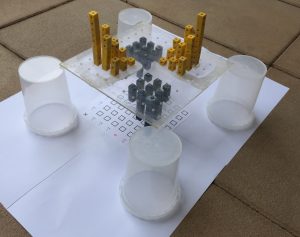
The Product Saddle
Like Number City, this is a large sculpture. The platform shown is 9 m on an edge and 4.5 m above the ground. The cubes of which the towers consist are 0.4 m on an edge. The platform supports are less bulky than shown in this mock-up. The platform itself is in a clear polymer, the towers of the same in the two colours suggested. The base is light concrete.
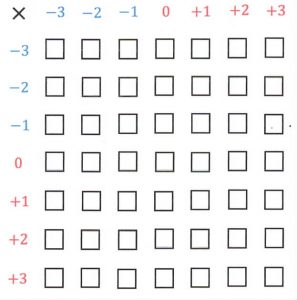
The sculpture realises in three dimensions part of the multiplication table for the integers, shown in two dimensions on the base. As shown in the inset, the products themselves are not specified. In a teacher-led session children can write them in with chalk. The point of the sculpture is to realise geometrically the algebraic symmetry of integer multiplication. Visitors uncertain why the product of two negative quantities should be positive can enjoy the fact as an aesthetic experience. Extended from the ring of integers to the field of real numbers, the surface is a hyperbolic paraboloid, a saddle, hence the name for the exhibit.
Look at these preparatory indoor tasks: